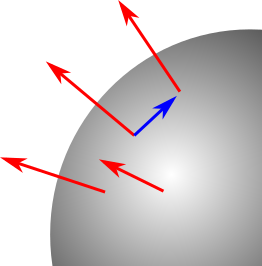
Suppose you have a spherically symmetric vector field, as in the diagram. Can we find an affine connection which transports the vectors into one-another? That is, a geometry in which they are all “parallel”?
Take Schwarzschild spacetime, in the usual coordinates ![]() . The coordinate basis vectors are
. The coordinate basis vectors are ![]() ,
, ![]() ,
, ![]() , and
, and ![]() . I will write these as
. I will write these as ![]() , so for
, so for ![]() for example, this is the vector
for example, this is the vector ![]() with components
with components ![]() . Recall a connection
. Recall a connection ![]() is defined by:
is defined by:
![]()
where the ![]() are the connection coefficients, also called Christoffel symbols in the specific case of the Levi-Civita connection. (Recall the Levi-Civita connection is the one inherited from the metric: it is the unique symmetric and metric-compatible connection.) For each pair
are the connection coefficients, also called Christoffel symbols in the specific case of the Levi-Civita connection. (Recall the Levi-Civita connection is the one inherited from the metric: it is the unique symmetric and metric-compatible connection.) For each pair ![]() , this definition is interpreted as the derivative of the
, this definition is interpreted as the derivative of the ![]() field, in the direction
field, in the direction ![]() .
.
Now consider an arbitrary vector field of the form:
![]()
We would not expect the sought-for parallel transport to work for vectors with components in the ![]() or
or ![]() -directions — at least, not without imposing extra choices. In particular, the “hairy ball theorem” states no smooth, non-vanishing vector field along the 2-sphere exists: that is, within its 2-dimensional tangent bundle. For Schwarzschild spacetime, we move around a 2-sphere of constant
-directions — at least, not without imposing extra choices. In particular, the “hairy ball theorem” states no smooth, non-vanishing vector field along the 2-sphere exists: that is, within its 2-dimensional tangent bundle. For Schwarzschild spacetime, we move around a 2-sphere of constant ![]() and
and ![]() , by taking “directional derivatives” along the
, by taking “directional derivatives” along the ![]() -plane. As expected,
-plane. As expected, ![]() does not vanish, even in these directions:
does not vanish, even in these directions:
![]()
The offending Christoffel symbols turn out to be ![]() and
and ![]() . These arise from
. These arise from ![]() and
and ![]() . These quantify how the radial coordinate vector changes as you move around on a sphere.
. These quantify how the radial coordinate vector changes as you move around on a sphere.
One option is to simply define new connection coefficients for which these vanish: ![]() and
and ![]() , and keep the remaining Christoffel symbols, in order to remain as close as possible to the metric connection. This procedure is justified, because given a frame field, any choice of smooth functions
, and keep the remaining Christoffel symbols, in order to remain as close as possible to the metric connection. This procedure is justified, because given a frame field, any choice of smooth functions ![]() yields a valid connection (Lee 2018
yields a valid connection (Lee 2018  , Introduction to Riemannian manifolds, Lemma 4.10). We can also write this new connection as the usual (Levi-Civita) covariant derivative plus a bilinear correction:
, Introduction to Riemannian manifolds, Lemma 4.10). We can also write this new connection as the usual (Levi-Civita) covariant derivative plus a bilinear correction:
![]()
The parenthetical term is a (1,2)-tensor we interpret as accepting the vectors in the last two slots (![]() in the second slot, and
in the second slot, and ![]() into the last), returning another vector. The correction term may also be written
into the last), returning another vector. The correction term may also be written ![]() , where the angle brackets mean contraction of a 1-form and vector in this case. Intuitively, the parenthetical term just above is also a projection, returning only the angular part of the differentiation direction
, where the angle brackets mean contraction of a 1-form and vector in this case. Intuitively, the parenthetical term just above is also a projection, returning only the angular part of the differentiation direction ![]() . This is the blue arrow in the original diagram. For large
. This is the blue arrow in the original diagram. For large ![]() , the basis vectors
, the basis vectors ![]() and
and ![]() grow very large, but the red
grow very large, but the red ![]() vectors must adjust only by the angle rotated through, hence the
vectors must adjust only by the angle rotated through, hence the ![]() multiplier.
multiplier. ![]() returns the radial component
returns the radial component ![]() .
.
As a check, ![]() as required. The new connection is not symmetric, because
as required. The new connection is not symmetric, because ![]() and
and ![]() remain non-vanishing. Hence the connection has “torsion”. I won’t write out its Riemann and Ricci tensors, but the scalar curvature is
remain non-vanishing. Hence the connection has “torsion”. I won’t write out its Riemann and Ricci tensors, but the scalar curvature is ![]() ! At face value this violates the Einstein field equations, for which the Ricci tensor (and hence the scalar curvature) always vanish in a vacuum, however Einstein’s equations use the Levi-Civita connection. Curiously, the value is precisely the scalar curvature for a 2-sphere.
! At face value this violates the Einstein field equations, for which the Ricci tensor (and hence the scalar curvature) always vanish in a vacuum, however Einstein’s equations use the Levi-Civita connection. Curiously, the value is precisely the scalar curvature for a 2-sphere.
We can also construct a symmetric connection ![]() for which additionally
for which additionally ![]() . In the (somewhat) index-free expression:
. In the (somewhat) index-free expression:
![]()
where ![]() is the symmetric product, and analogously for
is the symmetric product, and analogously for ![]() . This connection has Ricci tensor equal to the metric in the
. This connection has Ricci tensor equal to the metric in the ![]() and
and ![]() components, apart from a scalar factor
components, apart from a scalar factor ![]() , and vanishing elsewhere. Its scalar curvature is
, and vanishing elsewhere. Its scalar curvature is ![]() .
.
Hence we have constructed connections which parallel transport our spherically symmetric vector field around a sphere, and deviate as little as possible from the Levi-Civita connection. Neither of the new connections are “metric-compatible”, for instance ![]() . Hence
. Hence ![]() . The same holds for
. The same holds for ![]() .
.
If you find some formulae here do not work for you, compare your convention for the connection coefficient index order, or try swapping ![]() and
and ![]() in the correction terms. I had problems myself, so undertook a painstaking review of my own conventions, and wrote a new page describing them. Finally, beware of coordinate basis vectors! The “vectors”
in the correction terms. I had problems myself, so undertook a painstaking review of my own conventions, and wrote a new page describing them. Finally, beware of coordinate basis vectors! The “vectors” ![]() and
and ![]() actually depend on all four coordinates, which is related to the so-called “second fundamental confusion of calculus”! In case of ambiguity, perhaps some should be replaced with
actually depend on all four coordinates, which is related to the so-called “second fundamental confusion of calculus”! In case of ambiguity, perhaps some should be replaced with ![]() and
and ![]() , or scalar multiples thereof. I avoided this technicality in the interests of readability. This concern only applies to coordinate systems in which the metric is non-diagonal.
, or scalar multiples thereof. I avoided this technicality in the interests of readability. This concern only applies to coordinate systems in which the metric is non-diagonal.
One thought on “Affine connection for spherical symmetry”